
We have seen that tones are expressed with a slash in the form higher / lower. For example, the ascending tones of the Dorian mode are 1/1, 9/8, 32/27, 4/3, 3/2, 27/16, 16/9, and 2/1. Recall that an interval may be found by dividing a higher tone by a lower tone, and the answer is expressed with a colon in the form lower : higher. Any numbers may used to create a tone interval. Thus far we have introduced only two tone intervals, 8:9 and 243:256.

The names wholestep and halfstep refer to the way these intervals appear in a diatonic arrangement of natural tones.
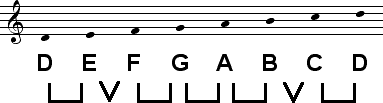
The form lower : higher is used to show the distance between a lower tone and a higher tone, as demonstrated when the scale above is read from left to right. Intervals occuring this way between successive tones are called a melodic intervals.

Tones which occur simultaneously also define intervals. Such an interval is called a harmonic interval. For harmonic intervals, the ratio may be written vertically in the form higher over lower, as shown below.

The idea of diatonic naturals expressed in staff notation provides a simpler way to represent intervals. Because the staff provides positions for notes indicating natural tones only, natural intervals are given names which simply correspond by the staff distances from one note to another. For example, the wholestep and the halfstep both span two staff positions, and are therefore both called Seconds, as shown below.

Intervals defined in this way may properly be called note intervals. Other note intervals are shown below.

Except for unisons and octaves, intervals are simply named by ordinal numbers. The word unison comes from the Latin word unisonus which literally means one sound, and the word octave literally means eighth. Below, harmonic intervals are shown for the Dorian mode.


Having established the concepts of melodic and harmonic intervals, we now return to the subject of modes.
NEXT: Harmonic Modes